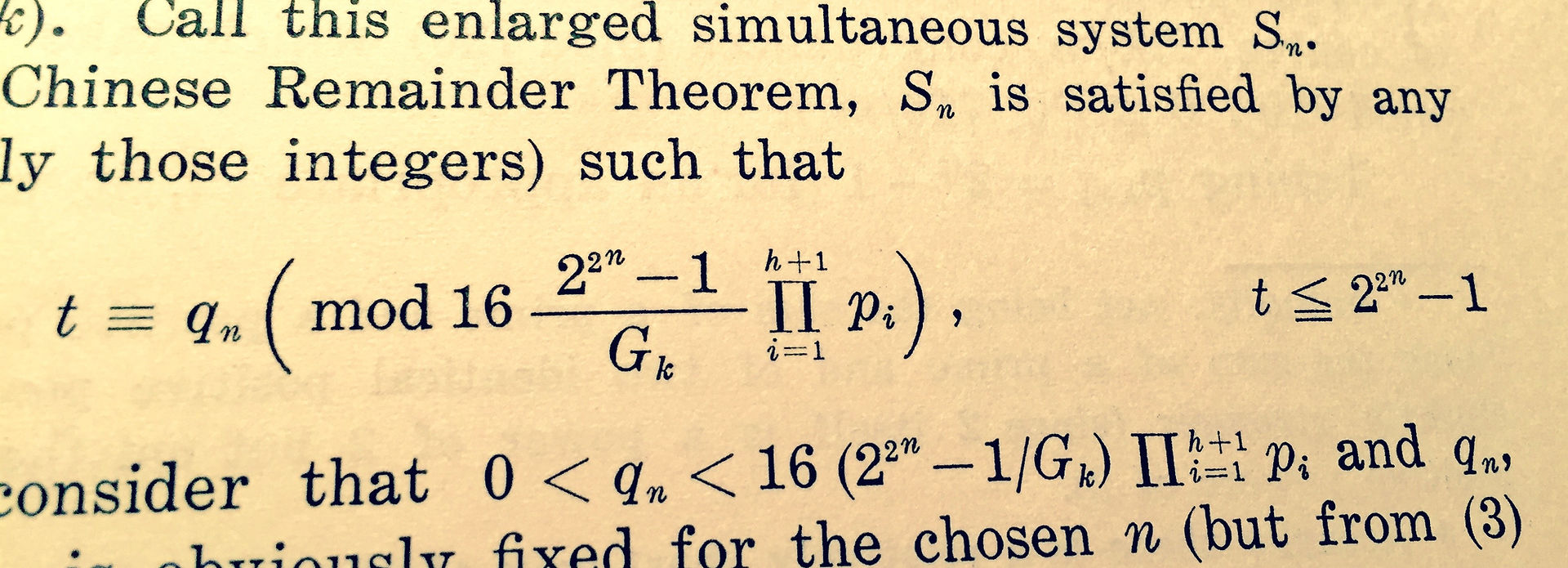
RESEARCHER in MATHS and PHYSICS
Roger Clement Crocker
ABOUT ME
Born and educated in the U.S.A., during which time Roger Crocker spent a year in Paris at a Lycee. He then obtained his Bachelors in Physics at Johns Hopkins University, U.S.A. and his Masters at Ohio State University, U.S.A. He was a lecturer in Mathematics at the University of Toledo, Ohio, U.S.A., as well as at John Carroll University in Ohio and Queen Elizabeth College, University of London, in the United Kingdom. For many years he has dedicated himself to independent research for which he has received recognition in a number of books.
He is married with a daughter and two grandchildren.

Mathematics Personal Comment
'A problem in Number Theory is as timeless as a true work of art' - David Hilbert
I think some comments on some of these articles are in order. The ones I would like to stress in Number Theory are [1], [8], [10], all dealing with what is called Additive Number Theory - whether all positive odd integers or even integers or both (as the case may be) can be represented as the sums of primes or powers or combinations of them.

A famous unsolved problem is whether every even integer > 2 can be expressed as the sum of two primes ( the Goldbach Conjecture).
Another (unsolved one) is whether every sufficiently large number can be written as the sum of four (non-negative) cubes. An important one, solved by U.V. Linnik, is proving that every sufficiently large integer is the sum of a prime and two squares. Combining (additively) primes with powers of 2 is another important one, and combining two squares with powers of 2 is yet another.
- The first of the three articles above [1] shows that there is an infinity of positive odd integers not representable as the sum of a prime and a power of 2; this had been proved previously by a totally different method - references are given in [1].
- The second of these articles [8] shows that there is an infinity of positive odd integers not representable as the sum of a prime and 2 (or fewer) powers of 2.
- The third of these articles [10] shows that there is an infinity of positive (even) numbers not the sum of two squares and two (or fewer) powers of k - for any fixed k ≥ 2 - the case k=2 is the most difficult and interesting. (There is also a Postscript making some further observations relating to [1] and [8].) These articles raise the questions of replacing two (or fewer) powers of 2 by three (or fewer) powers of 2 (in [8] and [10]). I suspect that if these questions are raised, either one could eventually prove that every sufficiently large positive odd integer or even integer (or both as the case may be) can be so represented, or these questions are undecideable. Obviously, if representablility can be proved, it would be well beyond the foreseeable techniques in Number Theory. (For further relevant information please here.)
Physics Personal Comment

As far [7] and [9], especially the first, a few points and opinions may be in order. There seems to be a feeling that the Uncertainty Principle (UP) is really more relevant to microscopic systems than to macroscopic ones - though UP does surface in Cosmology on occasion to try to explain the cosmic-microwave background fluctuations, and in some other ways that might seems rather questionable. I maintain that far greater use of UP in Cosmology is possible and that its full potential has not been realised (though trying to persuade some people of this seems difficult). My article [7], for which I received a number of requests for reprints, attempts to do just that, relating universal energy and momentum quantization throughout the universe at the smallest scale (using the UP) to the lifespan and size of the universe (if the latter two are finite). In a further article, which I am hoping to get published, I show how the UP - again in its basic and artificially unaltered form - can be used to get a good estimate of Planck time - and then go on to show, again, but in a slightly altered form, that the same kind of reasoning (as with Plank time) might lead to universal energy and momentum quantization throughout the universe at the smallest scale, and again might be intimately connected and expressible in terms of the lifespan and age of the universe - if indeed at least one of these is finite, which still must be considered at least as a definite possibility (see concept of 'quintessence'). The Editor of one journal would not pass it on to a referee, though [7] and [9] had already been published in his journal! The other claimed it was too 'speculative', which in light of some of the attempted, talked about, and published work in Theoretical Physics nowadays really is a case of 'look who's talking' - so much of this work is far more speculative and has been unable to acquire any experimental support whatsoever. Peter Woit, in his excellent book 'Not Even Wrong' has described much of the work published in some of the most prestigious Physics journals as 'egregious nonsense' (see Chapter 15).
In parts of Cosmology, there are almost as many theories as researchers, not exactly an auspicious situation. It is quite possible that some of these theories may never be verified - but cannot be proved false either - for by simply conveniently changing the parameters when expedient, they can be made to fit the experimental data. And when experiments fail to support a prediction or idea (e.g. proton decay or supersymmetry) excuses and contrived explanations are made to make the experimental failures look irrelevant and somehow to be ignored. Peter Woit's above-mentioned book points out the 'intellectual dishonesty' (his words on p228) often resulting in false assertions or impressions given to the public. And Lee Smolin's excellent book 'The Trouble with Physics' points out the tendency of so many scientists to follow a few leaders: most of them senior scientists are set in their beliefs and unwilling or unable to look at new ideas with any objectivity. Woit points out that some are incredibly presumptuous and arrogant. He goes on to add that often these 'leaders' use intimidation to promote their views (by their power over job applications and tenure prospects). Smolin seems to be of the same opinion. I might add that other outstanding physicists (Sheldon Glashow and Richard Feymann - quoted also by Smolin and Woit) have attacked superstring theory, in the strongest terms. And Woit (p206 in his book above) also comments on the unbelievable arrogance of string theorists. Smolin, on p282 of his same book points out that string theory may not really exist as a mathematical structure. Woit, on p181 of his above book points out that string theory 'isn't really a theory but rather a set of reasons for hoping that a theory exists' - and that this is the fundamental reason 'that string theory makes no predications'. Edward Witten, the guru of string theory, points out (1983) that 'what is really unsatisfactory at the moment about string theory is that it isn't yet a theory' - and both Woit and Smolin point out that this still remains the case today. Woit also, on p181, quotes an expert in the theory as saying 'M-theory is a misnomer. It is not a theory but a collection of facts and arguments which suggest the existence of a theory.' I could continue in this vein but I hope I have made clear the present situation in much of Theoretical Physics today. Both Smolin and Woit make some good suggestions about how to remedy this unfortunate situation, but I really wonder how much notice of them will be taken.... I have noticed that the better a suggestion is, the more likely it is to be ignored - and this is true in many other areas besides science.

RESEARCH INTERESTS
Number Theory
Please see my Blog on Number Theory.
Cosmology
Please see my Blog on Cosmology.